3차원 포물선
2022.12.12 - [ 업무관련] - 3D Parabola
3D Parabola
3D Parabola (3차원 포물선) 1. 가정 1-1. start point의 좌표(x1, y1, z1)는 (0,0,0)이다. 1-2. start point의 좌표(x1, y1, z1), End point(x3, y3, z3)에서 y1, y3은 0이다. 2. 엑셀에서 좌표를 계산한다. 3. 3점을 지나는 포물선
skyground21.tistory.com
https://en.m.wikipedia.org/wiki/Parabolic_coordinates
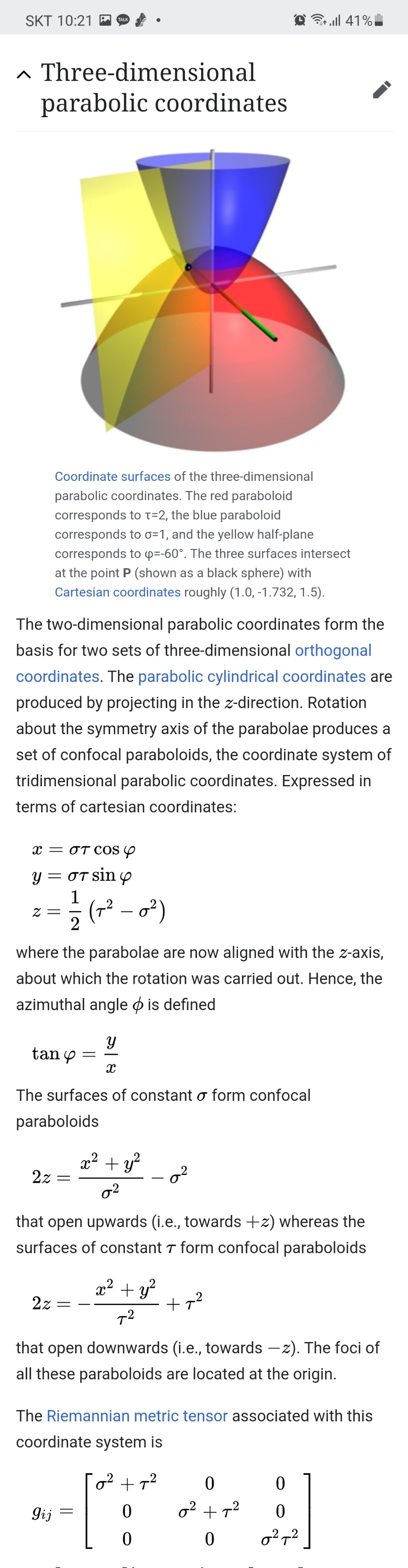
Parabolic coordinates - Wikipedia
Two-dimensional parabolic coordinates ( σ , τ ) {\displaystyle (\sigma ,\tau )} are defined by the equations, in terms of cartesian coordinates: x = σ τ {\displaystyle x=\sigma \tau } y = 1 2 ( τ 2 − σ 2 ) {\displaystyle y={\frac {1}{2}}\left
en.m.wikipedia.org
https://www.quora.com/How-does-a-3-dimensional-parabola-look-like-How-can-I-draw-it
How does a 3 dimensional parabola look like? How can I draw it?
Answer (1 of 2): How does a 3 dimensional parabola look like? How can I draw it? A 3 dimensional parabola is called a paraboloid. Its formula is z=\frac{x^2}{a^2}+\frac{y^2}{b^2}. As this is a three dimensional figure, it cannot be drawn exactly on a piece
www.quora.com
A parabola is a 2-dimensional curve and is never 3-dimensional. The corresponding 3-dimensional surface is called a paraboloid.
The surface obtained by rotating a parabola about its axis of symmetry is a particular case of an elliptic paraboloid. The sections of this surface by planes perpendicular to the axis of symmetry are ellipses, and those by planes containing the axis are parabolas. The standard form of equations of such surfaces is given by
(x/a)^2 + (y/b)^2 = 2z/c, which becomes a paraboloid of rotation referred to, above when a=b.
There are also hyperbolic paraboloids, whose standard sections are hyperbolas or parabolas. Their standard equations are (x/a)^2 -(y/b)^2 = 2z/c or xy=cz. These surfaces are saddle shaped (extended in all directions).
포물선은 2차원 곡선이며 결코 3차원이 아닙니다. 해당 3차원 표면을 포물면이라고 합니다. 대칭축을 중심으로 포물선을 회전시켜 얻은 표면은 타원 포물면의 특별한 경우입니다. 대칭축에 수직인 평면에 의한 이 표면의 단면은 타원이고 축을 포함하는 평면에 의한 단면은 포물선입니다. 이러한 표면의 방정식의 표준 형식은 다음과 같습니다. (x/a)^2 + (y/b)^2 = 2z/c, 이는 a=b일 때 위에서 언급한 회전 포물면이 됩니다. 표준 섹션이 쌍곡선 또는 포물선인 쌍곡선 포물면도 있습니다. 표준 방정식은 (x/a)^2 -(y/b)^2 = 2z/c 또는 xy=cz입니다. 이 표면은 안장 모양입니다(모든 방향으로 확장됨).





최근댓글